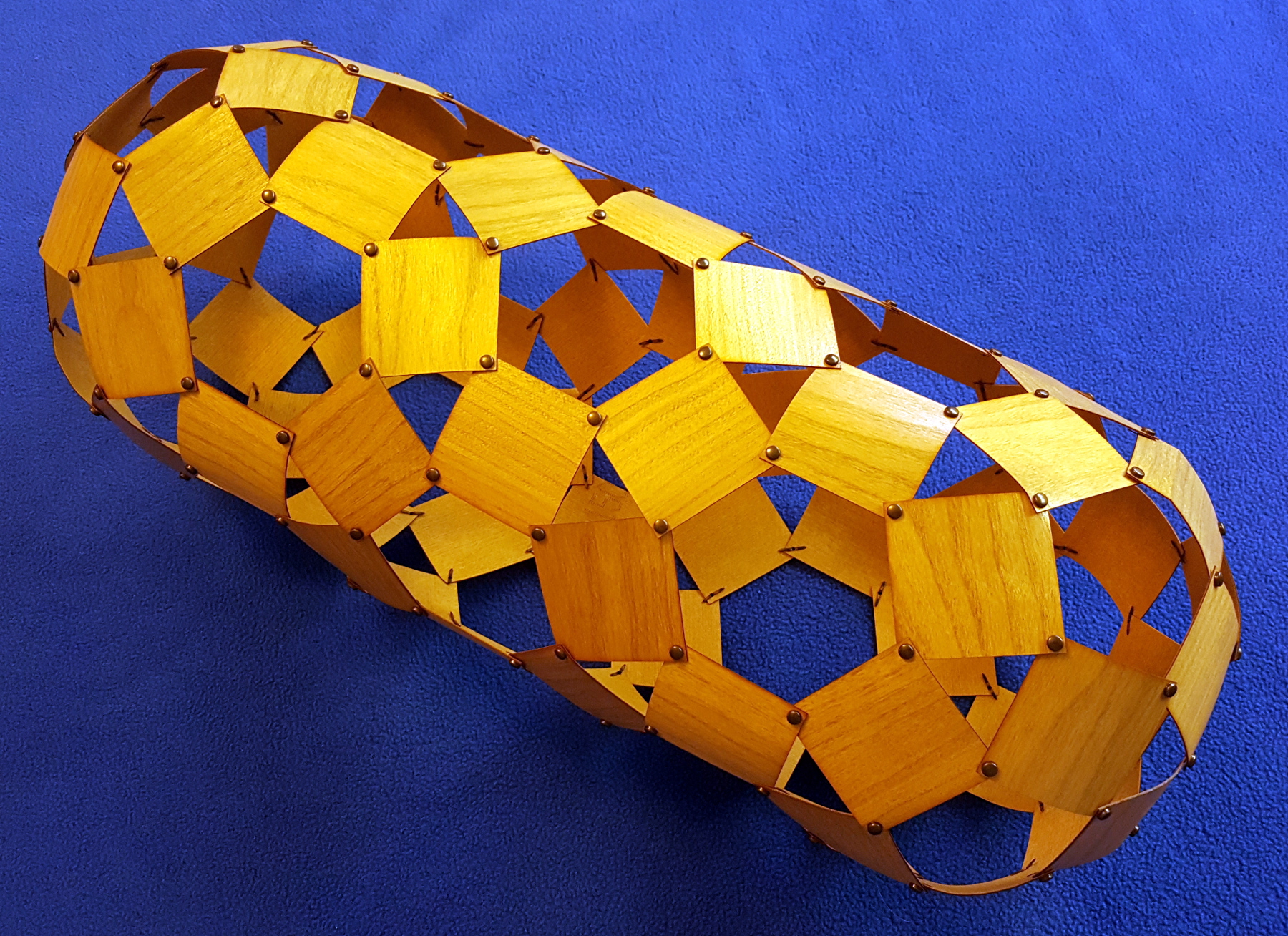
Capsule Cherry Veneer, 16 in 2017

This is a capped cylinder was created using 75 squares that are 5 cm on a side and connected at their corners using split-pin fasteners. The end-caps are based on dodecahedral hemispheres and the cylinder is based on a planar hexagonal tessellation, which results in a polyhedral form in which every vertex has order 3. The edges in the underlying form have been replaced by squares, resulting in an open lattice form. Every opening is either triangular (throughout), pentagonal (on the endcaps), or hexagonal (on the cylinder).

Related Works

• See the page on Expanded Polyhedral Forms.

Exhibition History

• Exhibition of Mathematical Art, Joint Mathematics Meetings, Atlanta, Georgia. 4–7 January 2017.

Publication History

• 2017 Joint Mathematical Meetings Exhibition of Mathematical Art, p. 69, Edited by Robert Fathauer and Nathan Selikoff, ISBN: 978-1-938664-21-2, Tessellations Publishing, 2017.

References

• Cogito, Ergo Summer by Siobhan Roberts. The New Yorker. 27 August 2015.
• Nonplanar expansions of polyhedral edges in Platonic and Archimedean solids, Bridges Conference Proceedings, July 2015. Paper (pdf)